A Sudoku Surprise
Once upon a time, I was a sudoku fiend. They provided an outlet, a distraction, a hiding place – I could bury my head in one for, say, half an hour and whatever had been troubling me before was somehow less of an issue.
It’s not an effective strategy for dealing with day-to-day living, but it was a useful tactic. (I remember being advised, at one point, that there wasn’t a global backlog I had to help sort out.)
And at some point, I got bored of them, or needed to hide less, and got out of the habit. I’m still interested in them mathematically, and when someone posted one on reddit with a question about how to solve it without guessing. It turned out, it sort of can - although it involves a fairly sophisticated shape I hadn’t seen used before.
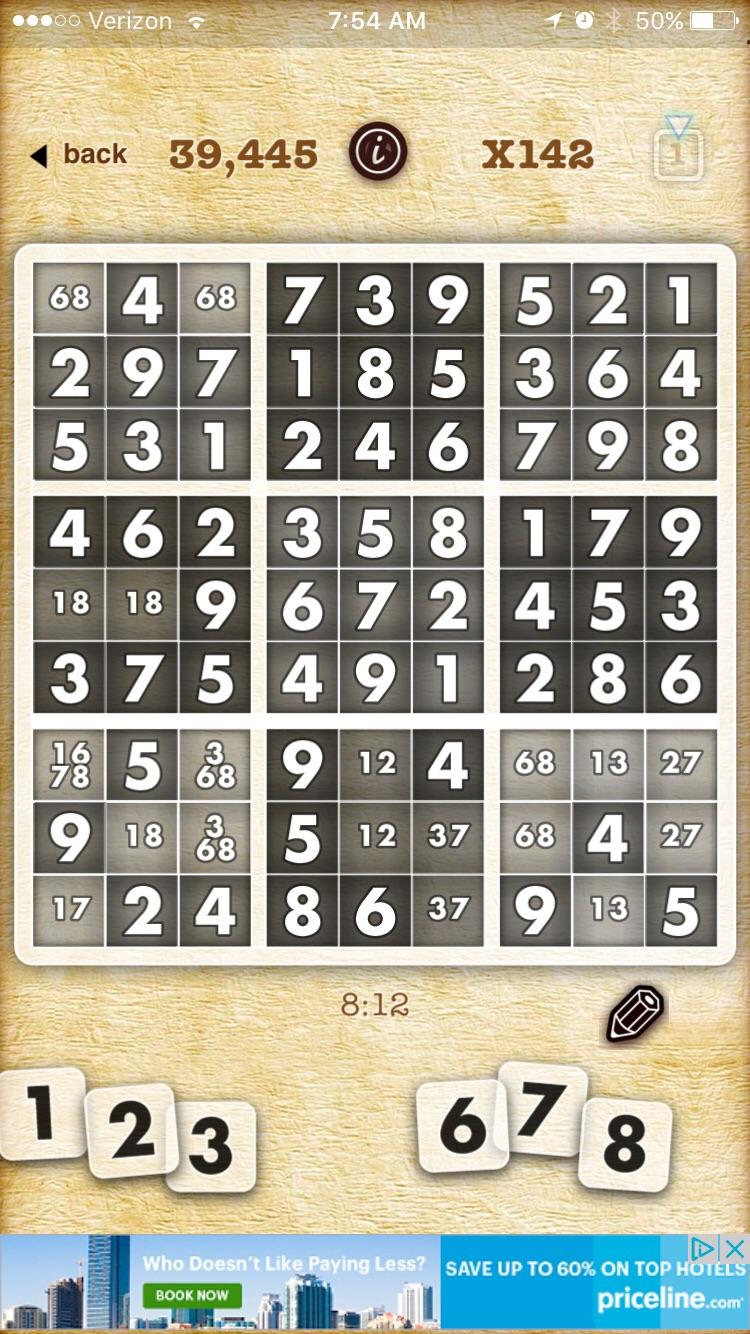
Here’s the grid - have a go, if you’d like to – there are spoilers below the line
The critical shape is in the two big squares in the bottom right, and it’s made up of four pairs of cells: in the bottom-middle square, the 12 pair and the 37 pair; in the bottom-right square, the 13 and 27.
There’s one extra bit of information I need to use: because of the 17 in the bottom left corner, one of the cells in the bottom row must be a 3.
That means the 37 in the middle line and the 13 in the top line can’t both be 3. (One of them must, however).
Now, let’s look at the 12 and 27 pair, which are arranged so that the 2s must be either in the top-left and bottom-right or top-right and bottom-left. BUT, in that second case, the opposite corners must be a 7 in the bottom-right and a 1 in the top-left - but that makes the middle 37 and top 13 both threes, which we can’t have!
So, in this scenario, the 1 must be below the 2 and the 7 must be above it. This situation gives no (direct) information about the two remaining pairs, although the rest of the grid falls apart once the 2s are in place.
Remaining questions
I have some questions about this.
- How do we spot such a pattern?
- How do we notate the grid so that such patterns are obvious?
- Is there a more succinct explanation of why the pattern works as it does?
I’d love to hear your thoughts in the comments!